Kelvin planck law deals with conservation of
Contents:
Kelvin-Planck’s law deals with
Considering reservoirs 1 and The thermodynamic temperature scale is also known as the Kelvin scale, and it needs only one fixed point, as the other one is absolute zero. The concept of absolute zero will be further refined during the statement of the third law of thermodynamics. Reservoirs are systems of large quantity of matter which no temperature difference will occur when finite amount of heat is transferred or removed.
Examples of reservoirs are atmosphere, oceans, seas etc. Clausius theorem states that any reversible process can be replaced by a combination of reversible isothermal and adiabatic processes. Consider a reversible process a-b. A series of isothermal and adiabatic processes can replace this process if the heat and work interaction in those processes is the same as that in the process a-b. Let this process be replaced by the process a-c-d-b , where a-c and d-b are reversible adiabatic processes, while c-d is a reversible isothermal process.
The isothermal line is chosen such that the area a-e-c is the same as the area b-e-d. Now, since the area under the p-V diagram is the work done for a reversible process, we have, the total work done in the cycle a-c-d-b-a is zero. Applying the first law, we have, the total heat transferred is also zero as the process is a cycle. Since a-c and d-b are adiabatic processes, the heat transferred in process c-d is the same as that in the process a-b. Now applying first law between the states a and b along a-b and a-c-d-b , we have, the work done is the same.
Thus the heat and work in the process a-b and a-c-d-b are the same and any reversible process a-b can be replaced with a combination of isothermal and adiabatic processes, which is the Clausius theorem. A corollary of this theorem is that any reversible cycle can be replaced by a series of Carnot cycles.
Suppose each of these Carnot cycles absorbs heat dQ 1 i at temperature T 1 i and rejects heat dQ 2 i at T 2 i. The negative sign is included as the heat lost from the body has a negative value. Summing over a large number of these cycles, we have, in the limit,.
Further, using Carnot's principle, for an irreversible cycle, the efficiency is less than that for the Carnot cycle, so that. As the heat is transferred out of the system in the second process, we have, assuming the normal conventions for heat transfer,. The above inequality is called the inequality of Clausius. Here the equality holds in the reversible case. Entropy is the quantitative statement of the second law of thermodynamics. It is represented by the symbol S , and is defined by.
Note that as we have used the Carnot cycle, the temperature is the reservoir temperature. However, for a reversible process, the system temperature is the same as the reversible temperature. Consider a system undergoing a cycle , where it returns to the original state along a different path. Since entropy of the system is a property, the change in entropy of the system in and are numerically equal.
Suppose reversible heat transfer takes place in process and irreversible heat transfer takes place in process Applying Clausius's inequality, it is easy to see that the heat transfer in process dQ irr is less than T dS.
Let us now consider the case of reversible engine for which. If you continue to use this site we will assume that you agree with it. However, it fails to say whether the processes or the cycle in a particular direction would occur at all or not. PACS Nos.: Joaquim Anacleto. We use cookies to ensure that we give you the best experience on our website. According to this statement, a system undergoing a cycle cannot develop a positive net amount of work from a heat transfer extracted from a thermal reservoir.
That is, in an irreversible process the same change in entropy takes place with a lower heat transfer. As a corollary, the change in entropy in any process, dS , is related to the heat transfer dQ as. This is called the principle of increase of entropy and is an alternative statement of the second law. Since T and S are properties, you can use a T-S graph instead of a p-V graph to describe the change in the system undergoing a reversible cycle.
Thus the area under the T-S graph is the work done by the system.
Further, the reversible adiabatic processes appear as vertical lines in the graph, while the reversible isothermal processes appear as horizontal lines. As a general rule, all things being equal, entropy increases as, temperature increases and as pressure and concentration decreases and energy stored as internal energy has higher entropy than energy which is stored as kinetic energy. From the second law of thermodynamics, we see that we cannot convert all the heat energy to work.
Question is ⇒ Kelvin Planck. is ⇒ Kelvin Planck's law deals with, Options are ⇒ (A) conservation of heat, (B) conservation of work, E. conservation of mass. Kelvin-Planck's law deals with (a) conservation of energy (b) conservation of heat (c) conservation of mass (d) conversion of heat into work Ans: d.
If we consider the aim of extracting useful work from heat, then only some of the heat energy is available to us. It was previously said that an engine working with a reversible cycle was more efficient than an irreversible engine.
Navigation menu
Now, we consider a system which interacts with a reservoir and generates work, i. Consider a system interacting with a reservoir and doing work in the process.
Suppose the system changes state from 1 to 2 while it does work. We have, according to the first law,. Since it is a property, it is the same for both the reversible and irreversible process. For an irreversible process, it was shown in a previous section that the heat transferred is less than the product of temperature and entropy change. Thus the work done in an irreversible process is lower, from first law. The availability function gives the effectiveness of a process in producing useful work.
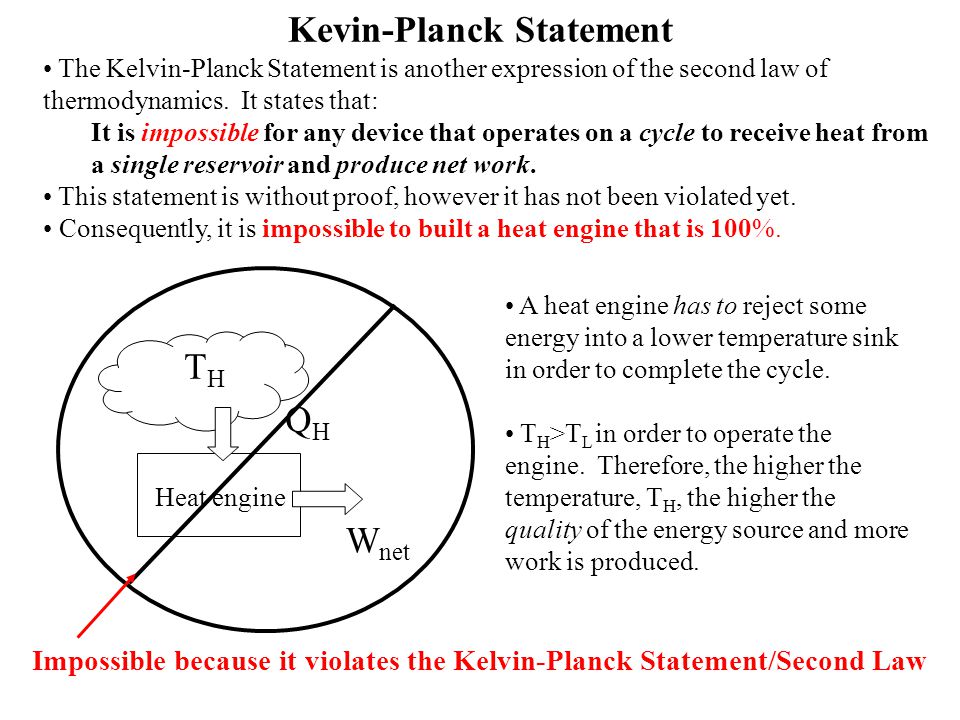
The Kelvin-Planck Statement
And yet there is an arrow to time! Even pendula slow down. Drop a ball and watch it bounce. Each bounce brings the ball not quite as high as the previous bounce.
General Physics II
A movie or a video of such a ball bouncing in the real world is different if viewed backward. Ice cubes dropped into a cup of hot tea melt while the hot tea becomes cold. It would be consistent with the First Law of Thermodynamics if we watched a cup of room temperature tea and found ice cubes forming while the remaining tea became hot and steam arose from it.
This is consistent with energy conservation. This scene would require no violation of energy conservation. But we never observe this! The Second Law of Thermodynamics will explain why or, at least, begin to!
- Kelvin–Planck statement?
- dominos pizza coupons duluth mn?
- coupon da stampare galbani.
- pizza pizza coupon march 2019.
The Second Law of Thermodynamics deals with the "arrow of time". Heat Engines Consider a heat engine that takes some "working substance" -- typically a gas -- through a cyclic process. Thermal energy Q h is absorbed from a source at a hot temperature T h. Net work W is done by the heat engine on its surroundings. Thermal energy Q c is given to a source at a cold temperature T c. This can be represented by diagram like this -- or We can also represent this process on a PV diagram.